Introduction to Nonlinear Optics and Its Quantum Impact

Nonlinear optics (NLO) is a branch of optics that deals with the interaction of light with matter in regimes where the response of the material is nonlinear. Unlike linear optics, where the optical properties such as refraction and absorption are independent of the light intensity, nonlinear optics describes scenarios where the optical properties change dramatically as the intensity of the incoming light increases [1].

Fundamentals of Nonlinear Optical Processes

In a linear optical medium, the polarization of the material (electric dipole moment per unit volume) is proportional to the electric field :

Here, is the linear susceptibility. However, at higher intensities, this relationship becomes nonlinear, and polarization can be expressed as:

In this expression, and are second- and third-order nonlinear susceptibilities, respectively, responsible for generating new frequencies or altering the properties of the incoming light [2].

Fundamentals of Nonlinear Optical Processes

In a linear optical medium, the polarization P
of the material (electric dipole moment per unit volume) is proportional to the electric field $E$ :

$P = ϵ_{0} χ^{(1)} E$

Here, $\chi^{(1)}$ is the linear susceptibility. However, at higher intensities, this relationship becomes nonlinear, and polarization can be expressed as:

P = \epsilon_0 (\chi^{(1)} E + \chi^{(2)} E^2 + \chi^{(3)} E^3 + \dots)

In this expression, $\chi^{(2)}$ and $\chi^{(3)}$ are second- and third-order nonlinear susceptibilities, respectively, responsible for generating new frequencies or altering the properties of incoming light [2].

Key Nonlinear Optical Processes

Second-Harmonic Generation (SHG): Conversion of two photons at frequency $\omega$ into one photon at frequency $2\omega$ .
Sum-Frequency Generation (SFG): Combining two photons at frequencies $\omega_1$ and $\omega_2$ to create a photon at frequency $\omega_1 + \omega_2$ .
Difference Frequency Generation (DFG): Producing photons at frequency $\omega_1 – \omega_2$ , crucial in quantum frequency conversion.
Optical Parametric Oscillation (OPO): Amplification and generation of new wavelengths by nonlinear interactions within a crystal. In an OPO, the pump photon splits into two lower-energy photons (signal and idler). Precise wavelength tuning is achieved by adjusting the crystal’s angle, thus affecting the phase-matching conditions [3].

The crystal orientation controls the effective refractive indices experienced by interacting waves, satisfying the phase-matching condition:

k_{\text{pump}} = k_{\text{signal}} + k_{\text{idler}}

Here, $k$ represents the wave vector, dependent on refractive index and frequency. Adjusting the crystal angle alters refractive indices, finely tuning output wavelengths [4].

Quantum Significance of Nonlinear Optics

Nonlinear optical phenomena play a pivotal role in quantum technologies. Particularly, Spontaneous Parametric Down-Conversion (SPDC), a special case of nonlinear frequency conversion, allows the generation of entangled photon pairs. These entangled photons form the foundational elements for quantum communication, quantum cryptography, quantum computing, and quantum-enhanced sensing [5].

Precise angular control of the crystal during SPDC directly influences the spatial geometry of the emitted entangled photons. Even minor angular misalignments affect the emission angles, pair correlations, and overall entanglement quality, emphasizing the importance of accurate crystal alignment in quantum experiments [6].

Nonlinear optics also facilitates quantum frequency conversion, enabling different quantum systems—such as quantum memories, quantum processors, and telecom communication channels—to interact efficiently [7].

Practical Challenges in Implementing Nonlinear Optics

Efficient nonlinear processes require precise phase matching, crystal alignment, temperature stabilization, and electrical modulation. Misalignment or temperature fluctuations can significantly reduce the efficiency and fidelity of quantum processes [8].

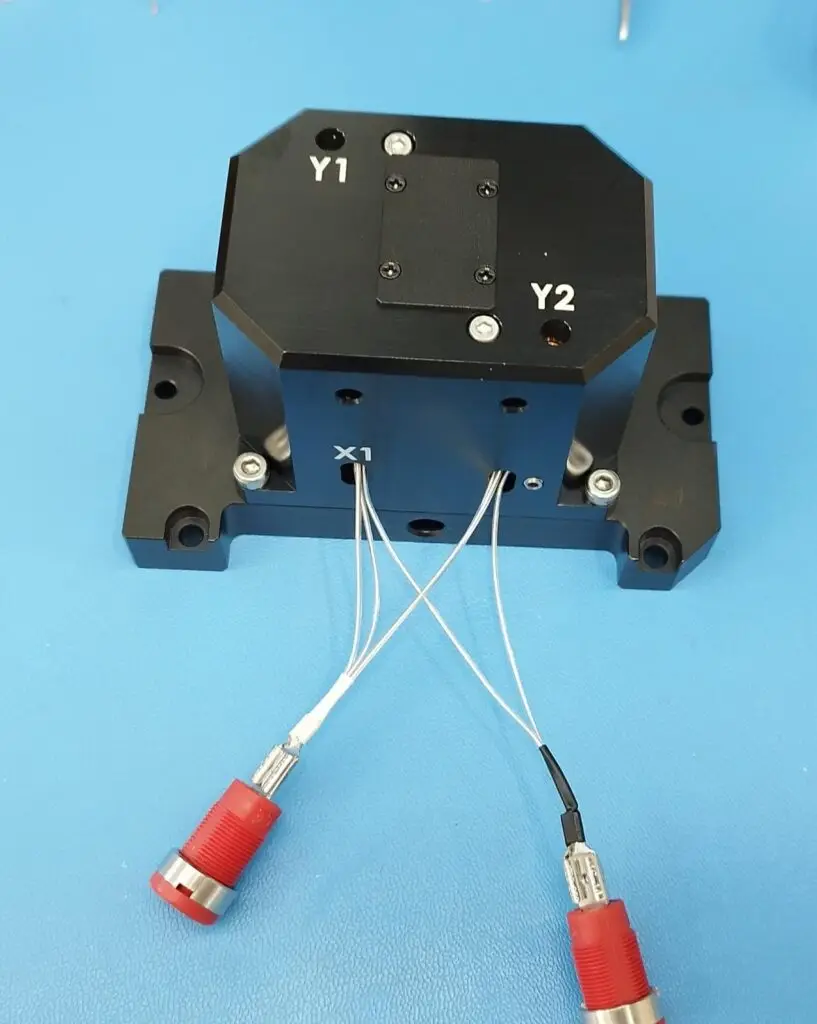
Optimizing Nonlinear Optics with IZAK NLO Kinematic Mount

To address these challenges, IZAK Scientific developed the IZAK NLO Kinematic Mount, designed explicitly for nonlinear optical applications. This mount provides:

Precise X and Y translation for accurate crystal positioning.
Controlled angular rotation about X and Y axes for precise phase matching.
Capability to apply high voltage directly to the crystal (useful in electro-optic modulation).
Integrated temperature control ensuring crystal stability and consistent nonlinear interactions.

By integrating these functionalities into one robust mount, the IZAK NLO Kinematic Mount significantly enhances the performance, reliability, and convenience of complex nonlinear quantum optical experiments and industrial quantum photonic systems.

Stay tuned as we continue exploring how nonlinear optics shapes quantum technology in future posts of our “Second Quantum Revolution” series.

References

[1] Boyd, R. W. (2008). Nonlinear Optics. Academic Press.

[2] Shen, Y. R. (2002). The Principles of Nonlinear Optics. Wiley-Interscience.

[3] Saleh, B. E. A., & Teich, M. C. (2007). Fundamentals of Photonics. Wiley.

[4] Dmitriev, V. G., Gurzadyan, G. G., & Nikogosyan, D. N. (1999). Handbook of Nonlinear Optical Crystals. Springer.

[5] Gerry, C., & Knight, P. (2005). Introductory Quantum Optics. Cambridge University Press.

[6] Kwiat, P. G., et al. (1995). New high-intensity source of polarization-entangled photon pairs. Physical Review Letters, 75(24), 4337.

[7] Kumar, P. (1990). Quantum frequency conversion. Optics Letters, 15(24), 1476-1478.

[8] Yariv, A., & Yeh, P. (2006). Photonics: Optical Electronics in Modern Communications. Oxford University Press.

Tzachi Sabati
CEO, IZAK Scientific  
Physicist specializing in photonics and quantum technologies, with deep expertise in quantum sensors and advanced optical systems. Leads the Advanced Quantum Lab course at the Technion, bridging academic excellence with industry innovation. At IZAK Scientific, provides cutting-edge photonics-based solutions, developing customized inspection and sensing systems for R&D and production. Passionate about advancing quantum sensing applications and integrating novel technologies to meet industry needs.